Find Inverse Of Matrix Using Row Operations
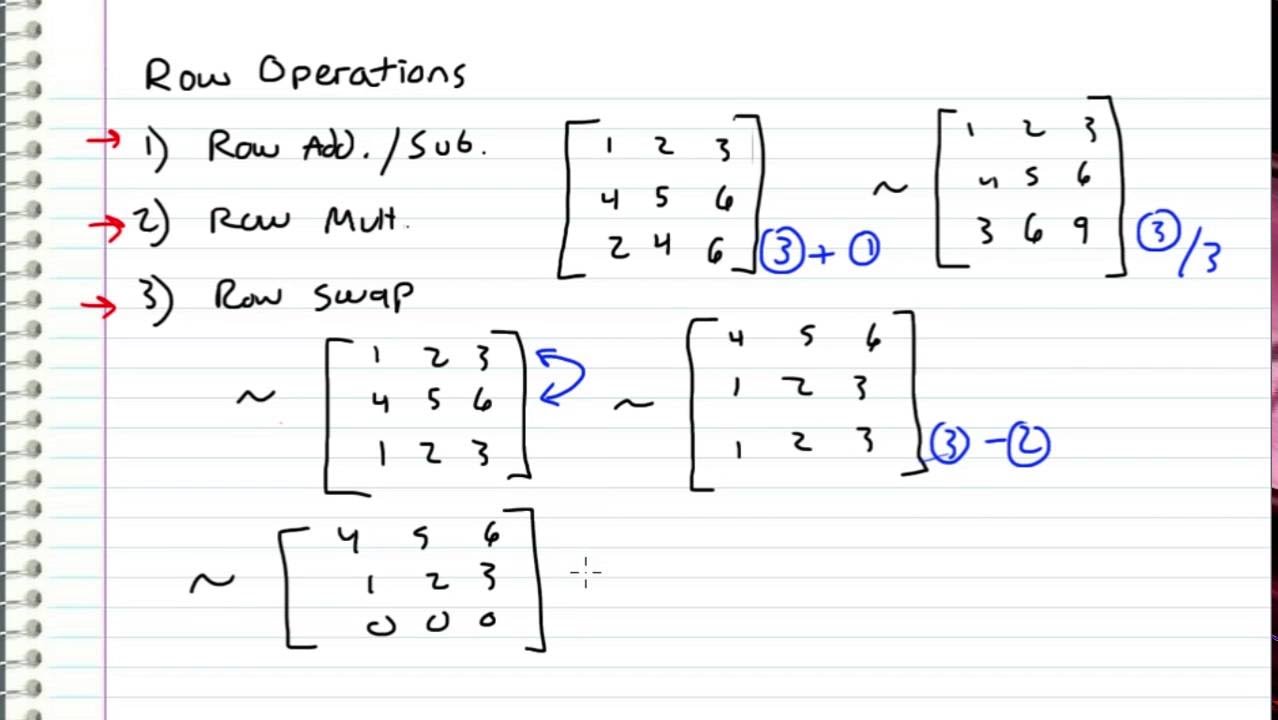
Matrix augmented inverse gauss jordan row operations using elementary called algebra mathsisfun Find row elementary transformation inverse using matrix matrices math question Inverse 3x3 matrices
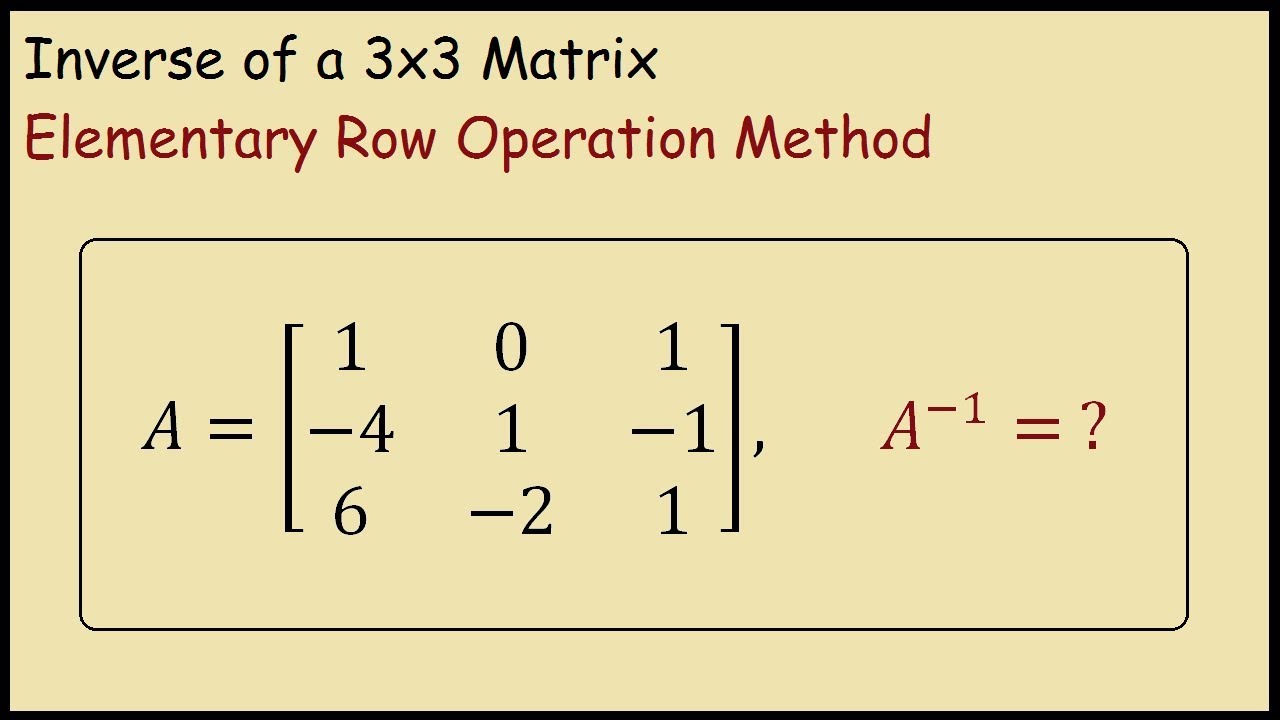
Using elementary row operations, find the inverse of the following
Inverse of a 3x3 matrix (using elementary row operations) Matrix inverse elementary using operations finding examples let take some Matrix inverse 3x3 row operations elementary using
Finding inverse of a matrix
Inverse elementary using matrix operations finding transformationMatrix inverse finding elementary using operations ex next Using elementary row transformation find the inverse of the matrix 3 -1Inverse matrices elementary.
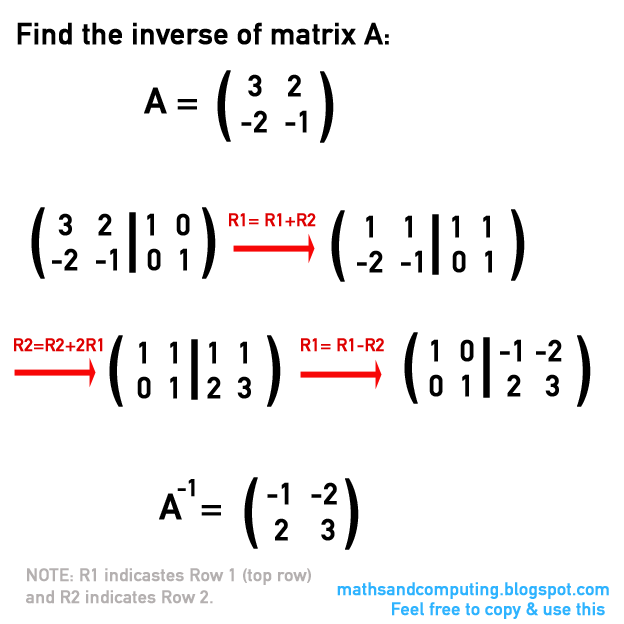
Maths and computing studies: finding the inverse of a matrixFind the inverse of the following matrix by using elementary row Find inverse of matrix using elementary row operation(ignore absence ofInverse sarthaks.

Finding inverse of a matrix
Matrix inverse 3x3 method find row elementary operation useElementary operations inverse matrix using finding Solved find the inverse of the following matrix by rowSolved using row operation find the inverse of the matrix.
Inverse of a matrix using elementary row operations (gauss-jordan)Using elementary row operations, find the inverse of the following Matrix elementary using find operation inverse row followingInverse of 3x3 matrices using row operations.
Matrix inverse
Matrix inverse finding example maths computing number right rowRow transformation inverse elementary find using matrix matrices Inverse 3x3 elementary row operations using matrices gauss jordan g1Find the inverse of a 3x3 matrix.
Linear algebraRow operations elementary algebra linear Matrix row inverse find reduction method following use technique question questions calculateFinding inverse of a matrix.

Finding inverse of a matrix
Using elementary row transformation find the inverse of the matrix 3 -1Row inverse matrix operation ignore absence bracket topperlearning 31st rashmi khot .
.